Exact Calculations of Nuclear Systems With Realistic Forces
A collaboration of Argonne National Laboratory, University of Illinois at Urbana-Champaign, Universidade de Lisboa, Los Alamos National Laboratory, Old Dominion University, and Thomas Jefferson National Accelerator Facility.
Constant density surfaces for a polarized deuteron in the Md = ±1 (left) and Md = 0 (right) states.
The deuteron, or 2H nucleus, contains one proton and one neutron and has a total angular momentum of 1. It can be oriented in a specific di"RECT"ion, for example by an external magnetic field, with possible spin projections of Md = +1 (parallel), -1 (antiparallel), or 0 (perpendicular). The force between two nucleons, which can be attributed to the exchange of pi-mesons at long range, has a strong tensor character which leads to these unusual shapes. The length of the dumbell and the diameter of the doughnut are both about 1.5 femtometer (1.5 x 10-15 meter).
Goals
We wish to understand the stability, structure, and reactions of nuclei as a consequence of the interactions between individual nucleons. In quantum mechanics, the state of a many-body system is described by its wave function, psi, while the motion and interaction of the particles is determined by the Hamiltonian. We are trying to build a consistent description of nuclear systems ranging in size from the deuteron to neutron stars using a single Hamiltonian. This requires finding accurate solutions to the many-body Schrödinger equation, se.
Problem
Realistic nuclear forces, which accurately describe nucleon-nucleon (NN) scattering and bound states, are very complicated. The most basic forces include central, spin-spin, tensor, and spin-orbit components, all with and without isospin dependence:
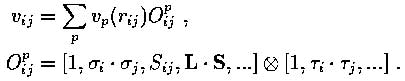
Even more components are required to get a really good description of NN data. We have constructed several force models over the years as new data has become available, and as the accuracy of the many-body calculations have improved. Our most recent force model, the Argonne v18 nucleon-nucleon potential 1, uses eighteen operator components to fit over 4,300 NN scattering data. There is also strong evidence for many-nucleon forces and special relativity can also be important. Solving the many-nucleon Schrödinger equation is consequently a very challenging theoretical problem.
Methods
We have been using quantum Monte Carlo (QMC) methods to study few-body nuclei. We start with a trial guess for the form of the wave function, and then systematically improve on it using the Green's function Monte Carlo (GFMC) algorithm to approach the true ground state:
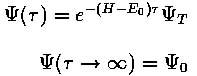
These calculations are computationally intensive, and our recent progress has required the use of massively parallel supercomputers. In fact, very rapid progress has been made in recent years, as detailed in the following table:
Progress in exact ground-state calculations
Year denotes the date when the first solutions accurate within 1% of the binding energy were obtained. FLOPS is the number of FLoating point OPerations required.
| Nucleus |
Method |
Year |
FLOPS |
Computer |
Time |
| 2H |
Diff Equation |
1953 |
50x103 |
Illiac-I |
15 min |
| 3H |
34-ch Faddeev |
1984 |
100x109 |
Cray XMP |
30 min |
| 4He |
GFMC |
1987 |
15x1012 |
Cray 2 |
40 hr |
| 5He |
GFMC |
1993 |
100x1012 |
Cray C90 |
100 hr |
| 6Li |
GFMC |
1995 |
300x1012 |
IBM SP1 |
6000 node-hr |
| 7Li |
GFMC |
1996 |
4x1015 |
IBM Sp2 |
1000 node-hr |
| 8Be |
GFMC |
1997 |
17x1015 |
IBM SP2 |
1300 node-hr |
To study larger systems, we use cluster variational Monte Carlo methods for closed-shell nuclei, and variational chain summation methods for nuclear and neutron matter.
Recent Progress
- QMC has been used to calculate the ground states and many low-lying excited states for all A<=8 nuclei 2,3,4, demonstrating for the first time the microscopic origin of nuclear shell structure. This is illustrated by the excitation spectra for A=6-8 nuclei.
- QMC calculations can explain both elastic and inelastic electromagnetic form factors observed in electron-scattering experiments, without the use of effective charges 5.
- The strong nuclear tensor force has been shown to produce novel toroidal correlations in nuclei6 as seen in the figure at the top of this page; several experiments have been proposed to search for these structures.
- Similar considerations lead to the prediction of pion-condensed phases in both nuclear and neutron matter near twice the saturation density, which has interesting implications for neutron star structure 7.
- The effects of special relativity on nuclear forces and nuclear binding are being studied in the framework of relativistic quantum mechanics8,9.
- QMC is being used to study neutron drops, collections of neutrons bound by an external well, thus providing bench marks for more schematic methods used to study nuclei far from stability and nuclei in the crusts of neutron star10,11.
- Studies have been made12 or are in progress for several radiative and weak capture reactions of astrophysical interest, including:
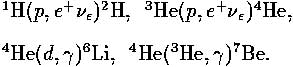
- The QMC wave functions are being used to study the feasibility of constructing polarized helium and lithium targets for electron-scattering experiments.
References
- Accurate nucleon-nucleon potential with charge-independence breaking, R. B. Wiringa, V. G. J. Stoks, and R. Schiavilla, Phys. Rev. C 51, 38 (1995).
- Quantum Monte Carlo calculations of A<=6 nuclei, B. S. Pudliner, V. R. Pandharipande, J. Carlson, and R. B. Wiringa, Phys. Rev. Lett. 74, 4396 (1995).
- Quantum Monte Carlo calculations of nuclei with A<=7, B. S. Pudliner, V. R. Pandharipande, J. Carlson, S. C. Pieper, and R. B. Wiringa, Phys. Rev. C 56, 1720 (1997).
- Quantum Monte Carlo calculations for light nuclei, R. B. Wiringa, Nucl. Phys. A631, 70c (1998).
- Microscopic calculation of 6Li elastic and transition form factors R. B. Wiringa and R. Schiavilla, Phys. Rev. Lett. 81, 4317 (1998)
- Femtometer toroidal structures in nuclei, J. L. Forest, V. R. Pandharipande, S. C. Pieper, R. B. Wiringa, R. Schiavilla, and A. Arriaga, Phys. Rev. C 54, 646 (1996).
- Spin-isospin structure and pion condensation in nucleon matter, A. Akmal and V. R. Pandharipande, Phys. Rev. C 56, 2261 (1997).
- Relativistic nuclear Hamiltonians, J. L. Forest, V. R. Pandharipande, and J. L. Friar, Phys. Rev. C 52, 568 (1995).
- Variational Monte Carlo calculations of 3H and 4He with a relativistic Hamiltonian, J. L. Forest, V. R. Pandharipande, J. Carlson, and R. Schiavilla, Phys. Rev. C 52, 576 (1995).
- Neutron drops and Skyrme energy-density functionals, B. S. Pudliner, A. Smerzi, J. Carlson, V. R. Pandharipande, S. C. Pieper, and D. G. Ravenhall, Phys. Rev. Lett. 76, 2416 (1996).
- Neutron drops and neutron pairing energy, A. Smerzi, D. G. Ravenhall, and V. R. Pandharipande, Phys. Rev. C 56, 2549 (1997).
- Weak capture of protons by protons, R. Schiavilla, V. G. J. Stoks, et al., Phys. Rev. C 58, 1263 (1998).